Calculations:Low Frequency Induction

Introduction

Low frequency induction (LFI) in metal pipelines is a form of electromagnetic interference that occurs when high voltage transmission lines are run in parallel with metallic pipelines.

The loaded phases on a transmission line and the pipeline act like single-turn windings on a large air-core transformer. Current flowing through the transmission line induces a voltage at the pipeline.

During normal operation, each loaded phase of the transmission line induces a voltage on the pipeline. If the three-phases are balanced, then most of the induced voltage will cancel each other out, but the spatial assymetry of the phases on the transmission line will prevent the induced voltages from fully cancelling out. Thus a non-zero induced voltage results on the pipeline. This condition is usually called "Load LFI".

During an earth fault condition, the phases of the transmission line are no longer balanced and as a result, a more significant voltage is induced on the pipeline. This condition is usually called "Fault LFI".

Why do the calculation?

LFI calculations are typically done for personnel safety reasons, in order to ensure that induced voltages are not hazardous to someone in contact with the pipeline.

In cases where such a situation is not possible, then an approach minimising the risk of electric shock could be followed. This could involve restricting access to the pipeline or using a probability-based methodology to estimate the risk of exposure, etc.

When to do the calculation?

The calculation should be done whenever HV transmission lines are installed in the vicinity of pipelines, and vice versa. Of particular concern is when the pipeline is run nearby and parallel to the transmission line for long distances. On the other hand, if a pipeline crosses the transmission line perpendicularly, then the magnitude of LFI would be low.

Calculation Methodology

The LFI calculation has nine general steps:

• Step 1: Data Gathering
• Step 2: Define the Zone of Influence
• Step 3: Define Pipeline Sections
• Step 4: Calculate Effective Distances
• Step 5: Calculate Pipeline Impedances
• Step 6: Calculate Mutual Coupling Impedances
• Step 7: Compute Load LFI
• Step 8: Compute Fault LFI
• Step 9: Calculate Pipeline-to-Earth Touch Voltages (if necessary)
• Step 10: Apply Mitigation Works (if necessary)

Step 1: Data Gathering

Before beginning the calculation, the following data needs to be gathered:

• Plan layouts of pipeline(s) and transmission line(s), to scale with enough resolution to measure horizontal distances between pipeline and transmission line
• General arrangement of transmission line tower or underground spatial arrangement
• Pipeline data, e.g. diameter, metal resistivity, coating details, etc
• Soil resistivity data around pipeline
• Forecast (or actual) load currents in transmission line
• Worst case prospective fault currents on transmission line

Step 2: Define the Zone of Influence

Based on the plan layouts of the pipeline and transmission line routes, the first step is to define the zone(s) of influence in which you will perform the LFI study. This zone is typically a corridor where the pipeline and transmission line are run close together in parallel.

As a general rule of thumb, the zone extends along this corridor until the horizontal separation between the pipeline and transmission line exceeds 1km. It is deemed that there is minimal LFI effect on the pipeline beyond a 1km pipeline-transmission line separation.

Step 3: Define Pipeline Sections

Once the zone of influence has been defined, the transmission line or pipeline should be broken up into small sections and the horizontal separations calculated for each section. The length of the indivudal sections do not have to be constant and can vary along the the route, depending on the rate of change of the horizontal separation.

For example, long section lengths can be applied when the pipeline and transmission line are roughly parallel. However, where the two lines converge, diverge or cross, smaller sectional lengths should be used (typically, a maximum ratio of 3:1 between the start of section and end of section separations is used to determine sectional length).

A table showing the sectional lengths and horizontal distances can then be developed, for example:

Section	Distance along pipeline (km)	Section Length (m)	Horizontal distance from phase A to pipeline (m)
			Start	End
1	0.0	300	140	190
2	0.3	200	190	200

Step 4: Calculate Effective Distances

The table we developed in Step 2 shows the horizontal separations between phase A and the pipeline at the start and end of each pipeline section. However, the transmission line will have three phases (and possibly two sets of conductors per phase) and the horizontal distances also do not take into account the geometry of the transmission tower.

Therefore, we'd like to further refine these distances. Ideally, we want a single effective distance between the pipeline and each phase (and if applicable, the earth wire). By using an effective distance, this makes it more convenient for us to calculate the induced voltages on a pipeline section.

This effective distance should incorporate the following:

• The average horizontal distance along the pipeline section
• The geometry of the transmission line towers (and the spatial geometry of the individual conductors on the tower)
• An equivalent distance for parallel conductors of the same phase

Transmission Line Geometry

Figure 1. Double conductor tower example

In order to construct the effective distances, we first need to define the spatial geometry of the transmission line with respect to the pipeline. This could be the geometry of transmission line towers or the spatial arrangement of underground conductors.

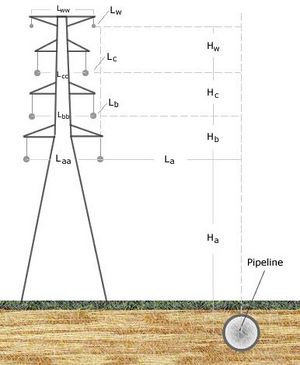
The figure to the right, showing a double conductor tower with two earth wires, gives an example of the kind of data that is required:

• L1 = Distance from pipeline to phase a
• L_b = Distance from phase a to phase b
• L_c = Distance from phase a to phase c
• L_w = Distance from phase a to the earth wire
• L_aa = Distance between phase a double conductors
• L_bb = Distance between phase b double conductors
• L_cc = Distance between phase c double conductors
• H_a = Height from pipeline to phase a
• H_b = Height from pipeline to phase b
• H_c = Height from pipeline to phase c
• H_w = Height from pipeline to earth wire

Of course your data requirements may vary depending on the transmission line type, but you need enough data to fully describe the spatial relationships between the phase conductors and the pipeline geometrically.

Effective Distances

As the horizontal distance between the transmission line and the pipeline is not necessarly constant along each section, we want to calculate an average horizontal distance for each line section. Typically, the geometric mean distance is used:

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle L_{m} = \sqrt{L1 \times L2} \, }

Where Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle L_{m} \, } is the effective horizontal distance between phase a and the pipeline

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle L1 \, } is the horizontal distance (between phase a and the pipeline) at the start of the section (m)

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle L2 \, } is the horizontal distance (between phase a and the pipeline) at the end of the section (m)

Similarly for double conductors arranged horizontally, we use the geomtric mean distance to obtain an effective distance between double conductors and the pipeline:

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle L_{a} = \sqrt{L_{m} \times (L_{m} + L_{aa}} \, }

Where Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle L_{a} \, } is the effective horizontal distance between double conductors (of phase a) and the pipeline (m)

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle L_{m} \, } is the effective horizontal distance calculated above (m)

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle L_{aa} \, } is the horizontal distance between the two conductors of phase a (m)

Note that the above show only horizontal distances. We can use simple Pythagoras to calculate the overall effective distance between phase a and the pipeline, taking into account the vertical arrangement of the conductors (relative to the pipeline). Below, we do this for a tower line:

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle D_{ap} = \sqrt{L_{a}^{2} + H_{a}^{2}} \, }

Where Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle D_{ap} \, } is the overall effective distance between the phase a and the pipeline

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle L_{a} \, } is the effective horizontal distance between double conductors (of phase a) and the pipeline (m)

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle H_{a} \, } is the height from the pipeline to phase a (m)

The same process above is repeated to calculate the effective distances for the other phases and the earth wire (if applicable).

The effective distances can be calculated also for other spatial conductor arrangements, using the geometric mean as a basis. Ultimately for each line section, we'd like to end up with only a single effective distance between the pipeline and a phase of the tower line.

Step 5: Calculate Pipeline Impedances

To assess the safety of a joint right-of-way installation, we want to calculate pipeline-to-earth touch voltages. However a buried pipeline cannot be treated like another overhead conductor in air because a pipeline has a finite impedance to earth that is distributed along its entire length.

Therefore the open-circuit voltages induced in the pipeline that we calculated earlier are not equivalent to the pipeline-to-earth touch voltage. There are continuous leakages to earth along the pipeline and the open-circuit induced voltages can be up to 10 times higher than the pipeline-to-earth touch voltages.

In order to calculate a more accurate pipeline-to-earth touch voltage, we need to consider the electrical characteristics of the pipeline.

The pipeline impedances described in this section are simplified approximations of Sunde's method [5] and are based on Appendix G of CIGRE Guide 95 "Guide on the Influence of High Voltage AC Power Systems on Metallic Pipelines" [2]. Refer to Sunde's original work [5] for more details on accurately modelling pipeline and other buried conductor impedances.

a) Pipeline longitudinal impedance

For a buried and coated metallic pipeline, the longitudinal impedance per metre can be approximated as follows:

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle Z_{p} = \frac{1}{\pi D} \sqrt{\pi f \rho_{p} \mu_{0} \mu_{p}} + \frac{\pi f \mu_{0}}{4} + j \left[ \frac{1}{\pi D} \sqrt{\pi f \rho_{p} \mu_{0} \mu_{p}} + \mu_{0} f \ln \left( \frac{3.7}{D} \sqrt{\frac{\rho}{\mu_{0} 2 \pi f}} \right) \right] \, }

Where Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle Z_{p} \, } is the pipeline longitudinal (or series) impedance (Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \Omega /m } )

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle D \, } is the diamter of the pipeline (m)

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \rho_{p} \, } is the resistivity of the pipeline metal (Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \Omega .m } )

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \rho \, } is the resistivity of the soil (Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \Omega .m } )

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \mu_{0} = 4 \pi \times 10^{-7} \, } is the permeability of free space (H/m)

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \mu_{p} \, } is the relative permeability of the pipeline metal (H/m)

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle f \, } is the nominal frequency of the transmission line (Hz)

b) Pipeline shunt admittance

For a buried and coated metallic pipeline, the shut admittance per metre can be approximated as follows:

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle Y_{p} = \frac{\pi D}{r_{c} \delta_{c}} + j \left[ 2 \pi f \frac {\epsilon_{0} \epsilon_{c} \pi D}{\delta_{c}} \right] \, }

Where Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle Y_{p} \, } is the pipeline shunt admittance (Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \Omega^{-1} /m } )

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle D \, } is the diameter of the pipeline (m)

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle r_{c} \, } is the resistivity of the pipeline coating (Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \Omega .m } )

$\delta _{c}\,$ is the thickness of the pipeline coating (m)

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \epsilon_{c} \, } is the relative permeability of the coating (H/m)

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \epsilon_{0} = 8.85 \times 10^{-12} \, } is the permittivity of free space (F/m)

The pipeline shunt impedance is simply the reciprocal of the admittance (remember that it is complex quantity):

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle Z_{p,sh} = \frac{1}{Y_{p}} \, }

c) Pipeline characteristic impedance

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle Z_{c} = \sqrt{\frac{Z_{i}}{Y_{i}}} \, }

Where Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle Z_{c} \, } is the pipeline characteristic impedance (Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \Omega } )

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle Z_{i} \, } is the pipeline longitudinal impedance (Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \Omega /m } )

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle Y_{c} \, } is the pipeline longitudinal conductance (Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \Omega^{-1} /m } )

d) Pipeline effective length

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle l_{e} = \frac{1}{ Re \left[ \sqrt{Y_{i} Z_{i}} \right] } \, }

Where Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle l_{e} \, } is the pipeline effective length (m)

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle Z_{i} \, } is the pipeline longitudinal impedance (Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \Omega /m } )

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle Y_{c} \, } is the pipeline longitudinal conductance (Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \Omega^{-1} /m } )

Step 6: Calculate Mutual Coupling Impedances

The mutual coupling impedance between a line conductor and the pipeline (with earth return) can be given by the general formula below (based on Carson's equations [4]):

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle Z_{lp} = \pi^{2} \times 10^{-4} f + j 4 \pi \times 10^{-4} \ln \left( \frac{D_{e}}{D_{lp}} \right) \, }

Where Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle Z_{lp} \, } is the mutual impedance between the line conductor Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle l \,} and pipeline Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle p \,} ($\Omega /km$)

$D_{lp}\,$ is the equivalent distance between the line conductor $l\,$ and pipeline $p\,$ (m)

$f\,$ is the nominal system frequency (Hz)

$D_{e}\,$ is the depth of equivalent earth return given by:

$D_{e}=658.37{\sqrt {\frac {\rho }{f}}}\,$

Where $\rho \,$ is the resistivity of the soil ($\Omega .m$)

Using the formula above, you can calculate the mutual impedances $Z_{ap}\,$, $Z_{bp}\,$ and $Z_{cp}\,$, between the pipeline and phase a, b and c of the transmission line respectively. To calculate the impedances in Ohms, multiply the mutual impedances by the sectional length of the pipeline.

Step 7: Compute Load LFI

Load LFI results from either unbalanced load currents or spatial differences between the phase conductors relative to the pipeline. Given the load current phasors for the transmission line, the total open-circuit induced LFI voltage on the pipeline is simply the vector sum:

$V_{p}=I_{a}Z_{ap}+I_{b}Z_{bp}+I_{c}Z_{cp}\,$

Where $V_{p}\,$ is the overall induced load LFI voltage (V)

$I_{a}\,$, $I_{b}\,$ and $I_{c}\,$ are the load current for phase a, b and c respectively (A). Note that these quantities are complex phasors

$Z_{ap}\,$, $Z_{bp}\,$ and $Z_{cp}\,$ are the mutual coupling impedances between the pipeline and phase a, b and c respectively for a section of the pipeline ($\Omega$)

Allowable Load LFI Limits

Induced voltages on pipelines represent are electric shock hazards. Because load LFI can be regarded as a continuous hazard (i.e. the hazard is always present), then the allowable pipeline-to-earth touch voltage limits are lower than in the fault LFI case. Limits are typically in the range of 32V to 64V (see the section on international standards below for more guidance on allowable limits).

Note that the open-circuit LFI voltage calculated earlier is higher than the pipeline-to-earth touch voltage as it doesn't take into account any voltage leakages to earth along the pipeline. Therefore, if the load LFI voltage you have calculated is lower than the allowable limits, then no further analysis is necessary.

Effect of Overhead Earth Wires and Counterpoise Earths

Overhead earth wires and counterpoise earth conductors provide a shielding effect (since a voltage is induced in them and they in turn induce an opposing voltage in the pipeline). The interaction of the overhead earth wires or counterpoise earth conductors with the pipeline can be modelled and a shielding factor computed (more on this later).

Step 8: Compute Fault LFI

During a balanced three-phase fault, the fault current will still be balanced across all three phases (i.e. vector sum of the faulted phase currents is close to zero). Therefore any induced voltage in the pipeline will be due primarily to the spatial assymetry of the transmission line relative to the pipeline, and this will generally be small.

However, an unbalanced fault such as a line-to-earth or line-to-line fault will produce a fault current that is unbalanced and can induce much higher LFI voltages on the pipeline (until the fault is cleared). The case of a line-to-earth fault will induce the highest LFI voltage and is the type of fault that will be examined hereafter.

The general formula for fault LFI is as follows:

$V_{f}=3I_{0}K_{sf}Z_{lp}\,$

Where $V_{f}\,$ is the overall induced fault LFI voltage (V)

$3I_{0}\,$ is the earth fault current, expressed as a complex phasor (A)

$K_{sf}\,$ is the shielding factor from earth wires, counterpoise earth conductors, etc

$Z_{lp}\,$ is the mutual coupling impedances between the pipeline and the faulted line for a section of the pipeline ($\Omega$)

Shielding Factor

The shielding factor is the shielding effect caused by earth wires, counterpoise earths and perhaps other metallic structures, which serves to lower the LFI voltage induced on the pipeline. There a number of mechanisms that bring about this shielding effect, for example:

• If there are overhead earth wires, then not all of the earth fault current will return to the source via the earth. Some of the fault current will return via the earth wires. The current flowing through the earth wire will induce a voltage on the pipeline opposed to the voltage induced by the faulted line.
• There is the interaction between the faulted line and overhead earth wires, where the faulted line induces a voltage on the overhead earth wires, which in turn induce an opposing voltage on the pipeline.
• There are similar inductive coupling interactions between the faulted line, the pipeline and counterpoise earths or other metallic structures located in the vicinity

For an overhead earth wire, the shielding factor can be calculated as follows:

$K_{sf}=1-{\frac {Z_{lw}}{Z_{ww}}}\times {\frac {Z_{wp}}{Z_{lp}}}\,$

Where $Z_{lw}\,$ is the mutual coupling impedances between the faulted line and the earth wire ($\Omega$)

$Z_{ww}\,$ is the self impedance of the earth wire ($\Omega$)

$Z_{wp}\,$ is the mutual coupling impedances between the pipeline and the earth wire ($\Omega$)

$Z_{lp}\,$ is the mutual coupling impedances between the pipeline and the faulted line ($\Omega$)

Considerations for Calculating Mutual Coupling Impedances

Calculating the mutual coupling impedances between the pipeline and the faulted line, $Z_{lp}\,$, depends on the phase of the faulted line and it's spatial orientation relative to the pipeline. You could calculate the mutual coupling impedance between the closest phase and the pipeline, which would represent the worst case scenario.

Alternatively, you could calculate the mutual coupling impedance for a group of conductors (e.g. all three phases) by considering the geometric mean separation distance between the pipeline and the group of conductors. This will result in an induced LFI voltage that is an average of the conductor group.

Similarly for groups of earth wires (e.g. two overhead earth wires), a geometric mean can be selected to represent the group rather than selecting a single wire, or modelling interactions between the wires, faulted line and the pipeline (which becomes increasingly complicated).

Allowable Fault LFI Limits

Like the case with load LFI, allowable limits for fault LFI are typically stipulated to prevent electric shock hazards from injuring or killing personnel. Because fault LFI is temporary (lasting only until the fault is cleared), the allowable limits are normally higher than in the load LFI case. The limits are generally based on some kind risk analysis and are typically in the order of 500V to 1000V (see the section on international standards below for more guidance on allowable limits).

Note that the open-circuit LFI voltage calculated earlier is higher than the pipeline-to-earth touch voltage as it doesn't take into account any voltage leakages to earth along the pipeline. Therefore, if the fault LFI voltage you have calculated is lower than the allowable limits, then no further analysis is necessary.

Step 9: Analysis of Pipeline-to-Earth Touch Voltages (if necessary)

If the open-circuit load or fault LFI voltage is above the permissible touch voltage, then the pipeline-to-earth touch voltages along each section of the pipeline need to be calculated. In order to calculate the pipeline-to-earth touch voltages, we need an equivalent circuit for the pipeline.

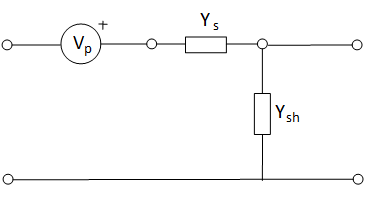
A common approach is to model the pipeline as a lossy transmission line, with the following equivalent circuit for each pipeline section:

Where $V_{p}\,$ is the induced LFI voltage on the pipeline section (Vac)

$Y_{s}\,$ is the longitudinal series admittance of the pipeline section ($\Omega ^{-1}$)

$Y_{sh}\,$ is the shunt admittance of the pipeline section ($\Omega ^{-1}$)

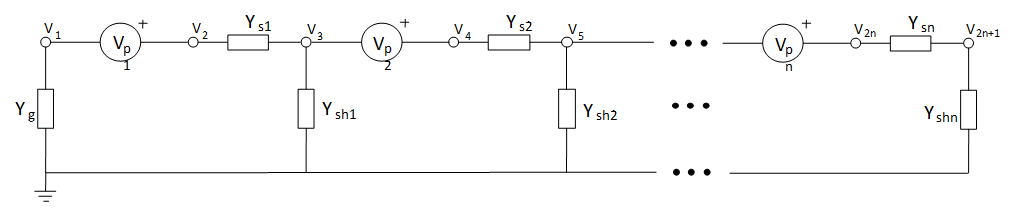
For a pipeline with "n" linear sections, the overall equivalent circuit model is therefore:

Where $Y_{g}\,$ is the admittance of a pipeline shunt earthing conductor ($\Omega ^{-1}$). The inverse of the pipeline characteristic impedance can be used if no shunt earthing conductors are installed at the ends of the pipeline. In the model above, shunt earthing conductors can be connected to any of the linear pipeline sections, modelled in series with the pipeline section shunt admittance.

The equivalent circuit can now be readily analysed using Kirchhoff's laws and converted into a system of linear equations. The unknown pipeline-to-earth node voltages can then be solved using matrix operations. Depending on the number of line sections, you will probably need to use a computer program to solve this linear algebra problem.

Step 10: Apply Mitigation Works (if necessary)

If the pipeline-to-earth touch voltages are still above the allowable limits, then the design of the right-of-way needs to be modified or mitigation works installed. Design modifications and mitigation works can include:

• Installation of shunt earthing conductors on the pipeline to underground earthing systems, to allow LFI voltage to drain to earth along sections of the pipeline
• Increasing the distance between the pipeline and the transmission line
• Installation of overhead earth wires or counterpoise earthing conductors
• Modifying the design of the pipeline, e.g. coating specification, diameter, etc
• Installation of gradient control wires alongside the pipeline, typically of zinc

International Standards

Most countries have their own standards for electromagnetic interference on pipelines.

In Europe, EN 50443:2011 is the standard for low frequency induction, conductive coupling and capacitive coupling. This has also been adopted by the British Standard BS EN 50443:2011.

In Australia, AS/NZS 4853:2000 stipulates the limits on pipeline-to-earth touch voltages. There are two main categories: Category A for pipelines with access to the public or unskilled staff, and Category B for pipelines with restricted access to skill personnel. For Category A, the load LFI limit is 32 Vac and fault LFI limit is between 32 and 350 Vac depending on the fault clearing time. For Category B, the load LFI limit is also 32 Vac, but the fault LFI limit is 1000 Vac (for faults cleared in less than 1s).

Computer Software

The LFI calculation is greatly simplified by using a computer software package, especially for more complicated joint rights-of-way. The following commercial software packages are among the most popular:

• SES Technologies CDEGS and in particular, the Right of Way module
• PRCI Pipeline A/C Interference & Mitigation Toolbox
• Elsyca IRIS

A free alternative for single pipeline and tower line rights-of-way:

• Sigma Power Low-Fi

What Next?

The LFI calculation is typically done to confirm the pipeline and / or transmission line design and to determine if mitigation is required. If mitigation measures are required, then the next step would be to design and specify these mitigation works.

Selected References

• [1] AS/NZS 4853:2000, "Electrical hazards on metallic pipelines"
• [2] CIGRE Guide 95, WG 36.02, "Guide on the Influence of High Voltage AC Power Systems on Metallic Pipelines", 1995
• [3] Schlabbach, J., "Short-circuit Currents (IET Power and Energy Series 51)", 2005, IEE
• [4] Tleis, N. D., "Power System Modelling and Fault Analysis", 2008, Elsevier Ltd
• [5] Sunde, E. D., "Earth Conduction Effects in Transmission Systems", 1968, Dover Publications
• [6] Carson, J., "Wave Propagation in Overhead Wires with Ground Return", 1926, Bell System Technology Journal, Vol 5, pp. 539-554